The fundamental theorem of Riemannian geometry and the Levi-Civita connection

The fundamental theorem of Riemannian geometry and the Levi-Civita connection November 10, 2009

Posted by Akhil Mathew in differential geometry, MaBloWriMo.
Tags: connections, Levi-Civita connection, Riemannian metrics
trackback

Ok, now onto the Levi-Civita connection. Fix a manifold ${M}$ with the pseudo-metric ${g}$ . This means essentially a metric, except that ${g}$ as a bilinear form on the tangent spaces is still symmetric and nondegenerate but not necessarily positive definite. It is still possible to say that a pseudo-metric is compatible with a given connection.

This is the fundamental theorem of Riemannian geometry:

Theorem 1 There is a unique symmetric connection ${\nabla}$ on ${M}$ compatible with ${g}$ .

The connection is called the Levi-Civita connection, even though Christoffel apparently discovered it first.

Recall that ${g}$ is compatible with ${\nabla}$ if and only if ${\nabla_X g = 0}$ for all ${X}$ . Since

$\displaystyle X(g(X_1,X_2)) = (\nabla_X g)(X_1,X_2) + g(\nabla_X X_1, X_2) + g(X_1, \nabla_X X_2) ,$

by the fact that covariant differentiation commutes with contractions and satisfies the derviative identity, compatibility is equivalent to

$\displaystyle X (g (X_1,X_2)) = g(\nabla_X X_1, X_2) + g(X_1, \nabla_X X_2),\ \ \ \ \ (1)$

for all ${X,X_1, X_2}$ .

Now assume ${M \subset \mathbb{R}^n}$ and we have such a connection associated to ${g}$ . Then the connection is uniquely determined by a bunch of Christoffel symbols, which we will determine in terms of ${g}$ by a bit of elementary algebra. In other words, we just need to compute ${\nabla_{\partial_i} \partial_j}$ . Now

$\displaystyle \partial_k g( \partial_i, \partial_j) = g( \nabla_{\partial_k} \partial_i, \partial_j) + g( \partial_i, \nabla_{\partial_k}\partial_j).$

We can get two other equations by cyclic permutation:

$\displaystyle \partial_i g( \partial_j, \partial_k) = g( \nabla_{\partial_i} \partial_j, \partial_k) + g( \partial_j, \nabla_{\partial_i}\partial_k)$

$\displaystyle \partial_j g( \partial_k, \partial_i) = g( \nabla_{\partial_j} \partial_k, \partial_i) + g( \partial_k, \nabla_{\partial_j}\partial_i)$

So let ${S_{ij} := \nabla_{\partial_i} \partial_j = \nabla_{\partial_j} \partial_i}$ , by symmetry. Let ${T_{ijk} := \partial_i g( \partial_j, \partial_k)}$ ; these are smooth real functions. These equations can be written

$\displaystyle T_{kij} = g( S_{ik}, \partial_j) + g( S_{jk}, \partial_i)$

$\displaystyle T_{ijk} = g( S_{ij}, \partial_k) + g( S_{ik}, \partial_j)$

$\displaystyle T_{jki} = g( S_{jk}, \partial_i) + g( S_{ij}, \partial_k)$

These are three linear equations in the unknowns ${g( S_{ik}, \partial_j), g( S_{jk}, \partial_i), g( S_{ij}, \partial_k)}$ . The system is nonsingular, so we get a unique solution, and consequently by nondegeneracy a unique possibility for the ${S_{ij}}$ .

We have just shown the uniqueness assertion of the theorem, which is local. Connections restrict to connections on open subsets.

Now for existence. Still with the hypothesis on ${M}$ , choose ${S_{ij}}$ to satisfy the system of three equations outlined above where ${i<j<k}$ . Then set ${S_{ji} := S_{ij}}$ , and we have a connection ${\nabla}$ with ${\nabla_{\partial_i} \partial_j := S_{ij}}$ since the vector fields ${\partial_i}$ are a frame (i.e. a basis at each tangent space on ${M}$ ). It is symmetric, since the torsion ${T}$ vanishes (by ${S_{ij}=S_{ji}}$ ) on pairs ${(\partial_i,\partial_j)}$ , and hence identically—since it is a tensor. Now to check for compatibility.

The difference of the two terms in (1) vanishes when ${X,X_1,X_2}$ are of the form ${\partial_i}$ . The vanishing holds generally because the difference of the two sides, which is ${(\nabla_X g)(X_1,X_2)}$ , is a tensor. Hence compatibility follows.

So we have proved the theorem when ${M}$ is an open submanifold of ${\mathbb{R}^n}$ (though not necessarily with the canonical metric ${\sum_{i=1}^n dx_i \otimes dx_i}$ ). In general, cover ${M}$ by open subsets ${U_i}$ diffeomorphic to an open set in ${\mathbb{R}^n}$ . We get connections ${\nabla_i}$ on ${U_i}$ compatible with ${g|_{U_i}}$ .

I claim that ${\nabla_i|_{U_i \cap U_j} = \nabla_j|_{U_i \cap U_j}}$ . This is an easy corollary of uniquness. So we can patch the connections together to get the one Levi-Civita connection on ${M}$ . In more detail, the whole idea of patching works as follows. Given ${X,Y}$ on ${M}$ and ${p \in M}$ , choose a neighorhood ${U}$ containing ${p}$ but contained in some ${U_i}$ . Multiply ${X,Y}$ by cutoff functions which are 1 close to ${p}$ but zero outside ${U}$ to get ${X',Y'}$ ; then set ${\nabla_X Y := \nabla_{i, X'}Y'}$ .

Comments»

1. Geodesics are locally length-minimizing « Delta Epsilons - November 13, 2009: […] Gauss lemma, geodesics, Riemannian manifolds trackback Fix a Riemannian manifold with metric and Levi-Civita connection . Then we can talk about geodesics on with respect to . We can also talk about the length of a […]

Reply
2. Ngô Quốc Anh - December 5, 2009: Aha, I think for the existence part, you can simplify as following: You have a metric g then you can define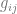 $g_{ij}$ , next you can construct $g^{ij}$ then we have Christoffel symbols $\Gamma_{ij}^k$ . So that we can define a connection $\nabla : TM \times TM \to TM$ as following

$\displaystyle\nabla_{\frac{\partial}{\partial x^i}}\frac{\partial}{\partial x^j}=\Gamma_{ij}^k\frac{\partial}{\partial x^k}$ .

Now we just verify that $\nabla$ is indeed a connection.

Reply
Akhil Mathew - December 5, 2009: Not sure how this is a simplification–after all, I described above how to compute the Christoffel symbols (just used $S_{ij}$ instead); locally, the Christoffel symbols can be arbitrarily prescribed for a connection.

Reply
3. Ngô Quốc Anh - December 5, 2009: For the uniqueness part, am I right that you have not proposed its proof? What we need is just the so-called “Vanishing Vector Fields” lemma.

Reply
Akhil Mathew - December 5, 2009: Uniqueness was checked locally because the Christoffel symbols were uniquely determined by the linear equations above. Hence, it’s true globally. What’s the vanishing vector fields lemma?

Reply
Ngô Quốc Anh - December 5, 2009: Any vector field that vanishes at $p$ can be written as a linear combination of other vector fields with coefficients that vanish at $p$ .
Akhil Mathew - December 5, 2009: To go from local to global uniqueness, you just need to multiply by a cutoff function. For instance, a connection on a manifold induces a connection on an open submanifold in a unique manner.
4. Identities for the curvature tensor « Climbing Mount Bourbaki - June 21, 2011: […] from a Riemannian pseudo-metric has to satisfy certain conditions. (As usual, we denote by the Levi-Civita connection associated to , and we assume the ground manifold is […]

Reply

	Evolution Gaming on The torsion tensor and symmetr…
	Proof that a group r… on Basics of group representation…
	Jahnke on The Hahn-Banach theorem and tw…
	pageanu on Some unsolved problems
	Ingo Blechschmidt on Generic freeness II

Delta Epsilons